|
|
Section 5.6
Designing Welds
Last Revised: 05/21/2023
The principle design variables for designing welds are:
- weld size (a for fillet welds),
- length (Lw),
- electrode type (FEXX), and
- the layout of the weld.
Another factor that affects weld strength is the strength of the base metal. However, for purposes of this discussion we assume that the connected parts have already been chosen so the applicable thicknesses and material strengths are fixed.
It is possible to have multiple weld sizes and segment lengths a weld group. There are an infinite number of combinations of the design variables.
The strength of weld group is based both on weld SIZE and weld LENGTH. Your choice of weld size will dictate the length of weld needed--the larger the weld, the shorter the required weld length.
The objective of the design is to ensure that you have the least cost weld that satisfies the applicable limit states--including size and strength limits.
Selecting Fillet Weld Size, a
It should be noted that in this text 'a' is used to designate leg size of fillet welds whereas in the SCM 'w' is used. Both notations are found in the literature.
Fillet weld size selection is limited by both strength and stipulated limits in the specification. When selecting a leg size, a, you should determine three different numbers:
- Minimum ALLOWED size
- Maximum ALLOWED size
- Largest EFFECTIVE size
The limit for MINIMUM ALLOWED SIZE was presented in BGSCM 5.3. The minimum allowed size is found in SCM Table J2.4.
The MAXIMUM ALLOWED SIZE is based on the geometric area available to accommodate the weld. The discussion on this topic can be found in BGSCM 5.3.
If the weld metal's strength exceeds the base metal strength, then increasing the weld's size further will not increase the strength of the connection. The weld size for which the weld base metal strength equals the base metal strength is known and the LARGEST EFFECTIVE size. This was discussed in BGSCM 5.3 and BGSCM 5.5. Rounding up to the nearest 16th of an inch results in the largest effective weld size.
The weld size, a, then must satisfy the following criteria:
min allowed size < a < min[max allowed size, largest effective size]
When selecting the weld size, you should be aware that any fillet weld with a leg size, a, over 5/16" will require a minimum of three passes to complete. This adds substantially to the cost of the weld. If you have the choice (i.e., not limited by the above equations or by connection geometry) choose fillet welds that are not larger than 5/16".
Note, selecting weld size will simply give you the strength per unit length of the welded connection. The problem is not complete until you configure the weld and compute the capacity of the connection.
Concentrically Loaded Weld Groups
When designing weld groups, it can be advantageous to design the weld layout such that the center of resistance of the welds aligns with the center of force of the connected member. Doing so prevents the need to analyze the welds as eccentrically loaded weld groups. A weld group whose center of resistance aligns with the center of force is said to be "balanced".
Note that experience and research has shown that balancing welds is usually not necessary for single and double angle members due to the very small eccentricities involved (See SCM J1.7). It would appear that the assumption that allows you to neglect balancing is that there are parallel welds on either side of the faying surface which result in a small eccentricity between the center of gravity of the weld group and the center of gravity of the angle section. By doing enough eccentrically loaded connection computations it is easy to show that the practice of ignoring weld balancing for single and double angle members is justified as long as the eccentricities are kept small. Regardless, the basic principles for designing balanced welds are presented here on the chance that you may encounter a situation where the eccentricity is significant.
To determine the center of resistance of a weld group we use principles of statics.
- The sum of forces (i.e., resistance of the weld group) equals the applied force, in the direction of the applied force.
- The sum of moments (due to the applied force and resistance of the welds) in the plane of the faying surface is made to equal zero.
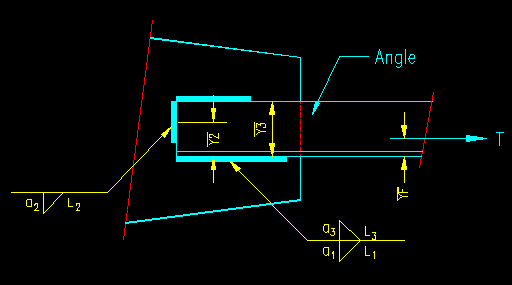
Consider the connection shown in Figure 5.6.1.
Figure 5.6.1
Welded Connection
Click on image for larger view
This connection is made with three fillet welds as shown. Each weld, potentially, has a different weld size and a different length. Each weld provides a portion of the resistance to force T as shown in Figure 5.6.2.
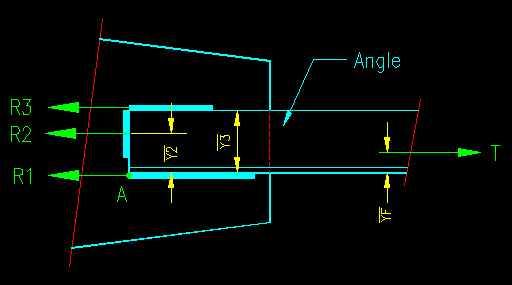
Figure 5.6.2
Weld Forces
Click on image for larger view
The relative magnitudes of the weld forces can be expressed in relation to their capacities.
R1 = Fnv te1 L1 = 0.60FEXX
(0.707a1) L1
R2 = Fnv te2 L2 = 0.60FEXX (0.707a2)
L2
R3 = Fnv te3 L3 = 0.60FEXX (0.707a3)
L3
The resultant reaction is
RT = R1 + R2 + R3
The eccentricity of the force to the weld group will be the perpendicular distance between the applied force T and the resultant reaction RT.
To eliminate the eccentricity, the forces must be colinear. That is the forces act upon the same line of action. Relative point "A" in Figure 5.6.2, this means that YF equals the dimension from "A" to the line of action of RT. Using the terms defined in Figure 5.6.2, the balanced condition occurs when:
![]()
Substituting in the expression for R1, R2, and R3:
![]()
Assuming the same electrode for all welds, the expression simplifies to:
![]()
If all the welds are the same size (i.e., a1= a2 = a3), then all the leg size terms can be eliminated as well.
The objective of the weld layout design now is to select weld sizes (a1, a2, and a3) and lengths (L1, L2, and L3) such that the center of the reaction force (i.e., the right side of the equation) equals the center of the applied force and the strength of the weld group exceeds the demand.
Eccentrically Loaded Weld Groups
Eccentricity may be either in plane with the weld group, out of plane of the weld group, or both.
The design of eccentrically loaded weld groups is primarily a trial-and-error operation. For every combination of design variables considered an analysis using methods of BGSCM 5.2 must be performed to determine the internal forces on the weld group. The worst-case force per unit length is then compared to the weld capacity.
If there is significant reserve capacity, then the designer might choose to reduce the amount of weld and try another combination.
If the weld does not provide sufficient strength, then either additional weld is needed, or the configuration of the welds may be changed to get acceptable results.